Earthquakes are a physical process that releases elastic strain energies in the Earth’s crust by shear faulting. The elastic strain energy is a function of stress fields. Therefore, to understand earthquake generation, it is important to know stress fields and fault strength. However, these physical quantities are not well understood at present mainly because of difficulty in measuring the stress state in situ. I aim to estimate these quantities based on theoretical considerations, data analysis and seismic observations.

• Tectonic stress fields
• Pore-fluid pressure fields
• Interaction between earthquakes and volcanoes

Tectonic stress fields

The Japanese islands are located in very complex tectonic setting where Pacific, Philippine Sea, North America and Eurasian plates interact with each other. In and around Japan, a variety of seismic activities are observed both temporally and spatially, reflecting the complicated stress fields caused by the tectonic environments. After the 1995 Hyogo-ken Nanbu earthquake, dense seismic observation networks were established throughout Japanese islands, which enabled us to obtain comprehensive focal mechanism/moment tensor data not only for large earthquakes but also for small to medium earthquakes. Applying an original inversion method to the large amount of high-quality data, we successfully estimated the 3-D tectonic stress fields that illuminate the present-day (Quaternary) complex tectonic motion of Japanese islands.

The stress types of the Kuril–Japan–Nankai arc are basically E–W compression, but the direction of intermediate principal stress changes from N–S (reverse faulting type) in northeast Japan to vertical (strike–slip faulting type) in southwest Japan. On the other hand, the stress type of the Ryukyu and Izu–Bonin back arc regions is basically trench perpendicular tension (normal faulting type). In addition to these basic stress patterns governed by mechanical interaction between the four plates, we can recognize several characteristic local stress patterns corresponding to the horizontal motion of the Kuril fore–arc sliver, the collision of the Izu Peninsula with the mainland of Japan, and the opening of the Beppu–Shimabara rift zone.

Thus, although it is possible to estimate the types of stress fields (directions of the three principal stress axes and relative ratio of principal stresses) from a large amount of earthquake data, the actual level of stress (absolute values of principal stresses) is not well understood. The responses of stress fields to changes caused by natural phenomena, such as large earthquakes, provide clues for addressing the issue. Knowing the absolute stress level acting in the crust is an essential issue in seismology.

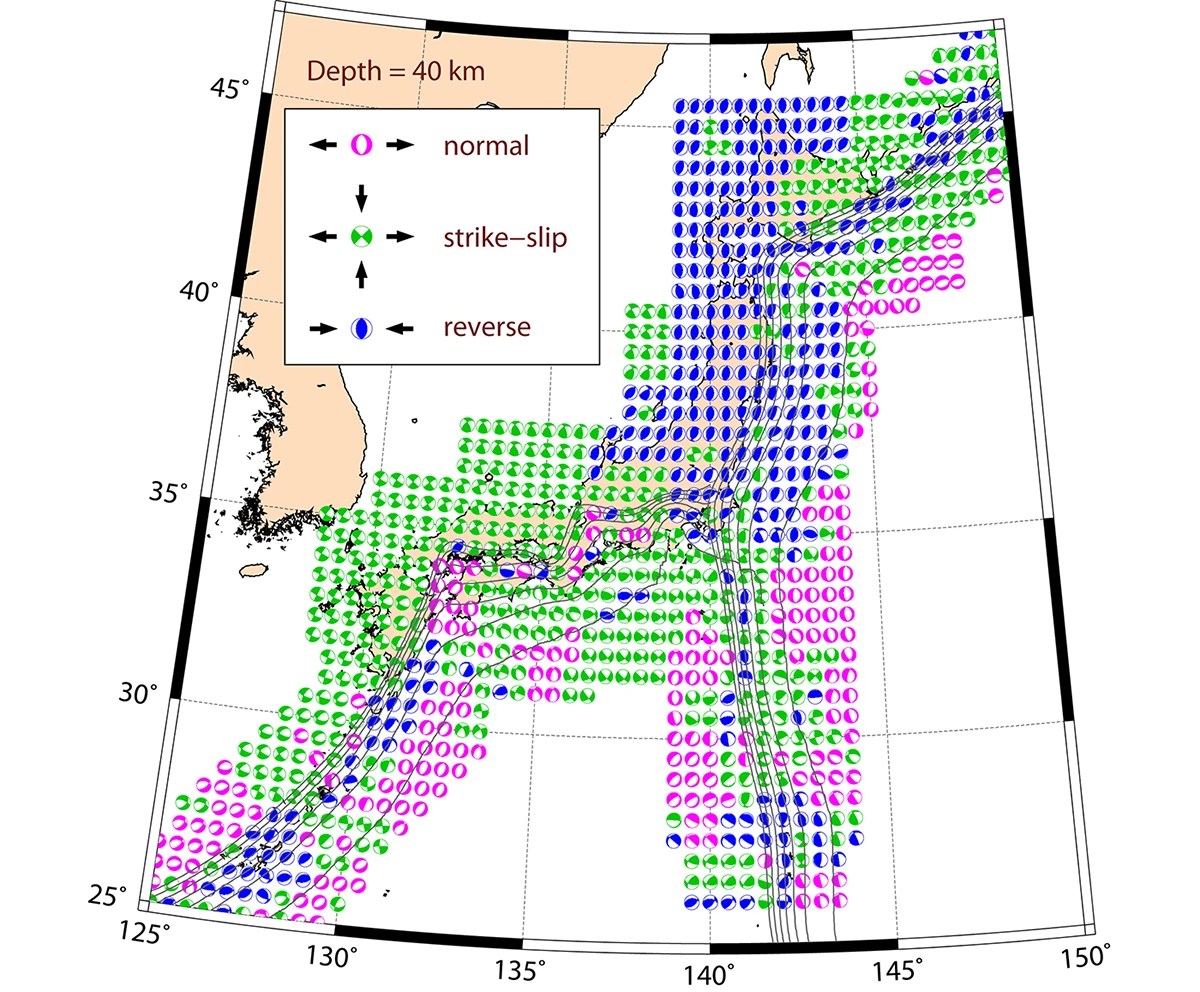
Fig. 1: Tectonic stress field in and around Japan (Depth = 10km).

The stress orientations are represented with the lower hemisphere projections of focal spheres which show shear stresses acting on the maximum shear stress planes at each location.

Pore-fluid pressure fields

Earthquakes are controlled not only by the stress field, but also by the strength of faults. Over-pressurized fluids trapped in the fault zones reduce the fault strength by way of decreasing effective normal stresses, which can trigger earthquakes. We have developed an inversion method to estimate the 3-D pore-fluid pressure field in the subsurface by examining the relationship between the focal mechanism data and the stress field. Applying the method to seismicity induced by fluid injections in the Basel enhanced geothermal system (EGS) in Switzerland, we successfully reproduced the spatiotemporal evolution of pore-fluid pressure in the reservoir in detail. The analysis shows that many of the earthquakes induced by injection experiments were caused by a decrease in fault strength due to an increase in pore fluid pressure. In natural earthquakes, it is very important to understand the role of stress and pore fluid in the generation of earthquakes.

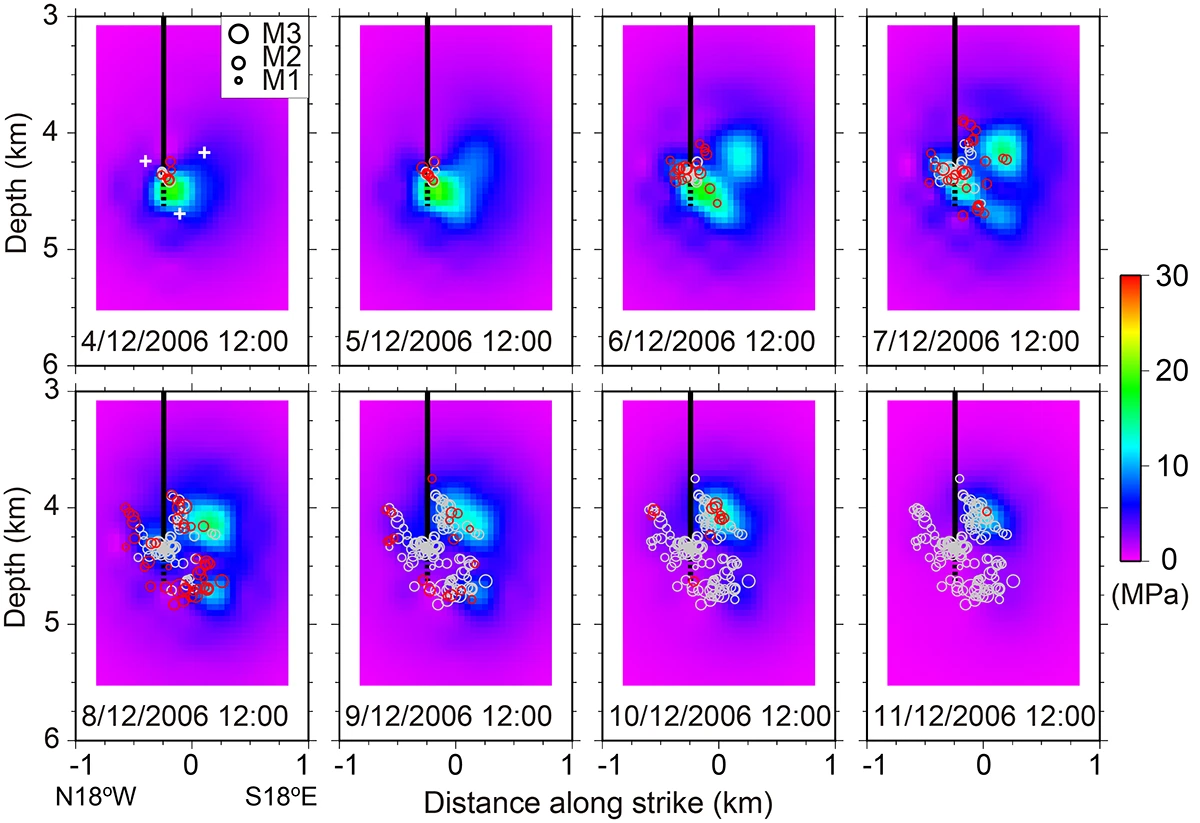
Fig. 2: Spatiotemporal evolution of pore-fluid pressure in the Basel EGS.

The color scales denote the excess pore-fluid pressure over hydrostatic. The black line denotes the injection well, and the dotted part denotes the open hole section. The red and gray circles denote seismic events within the time interval half day before and after the time indicated in each panel and the earlier events.

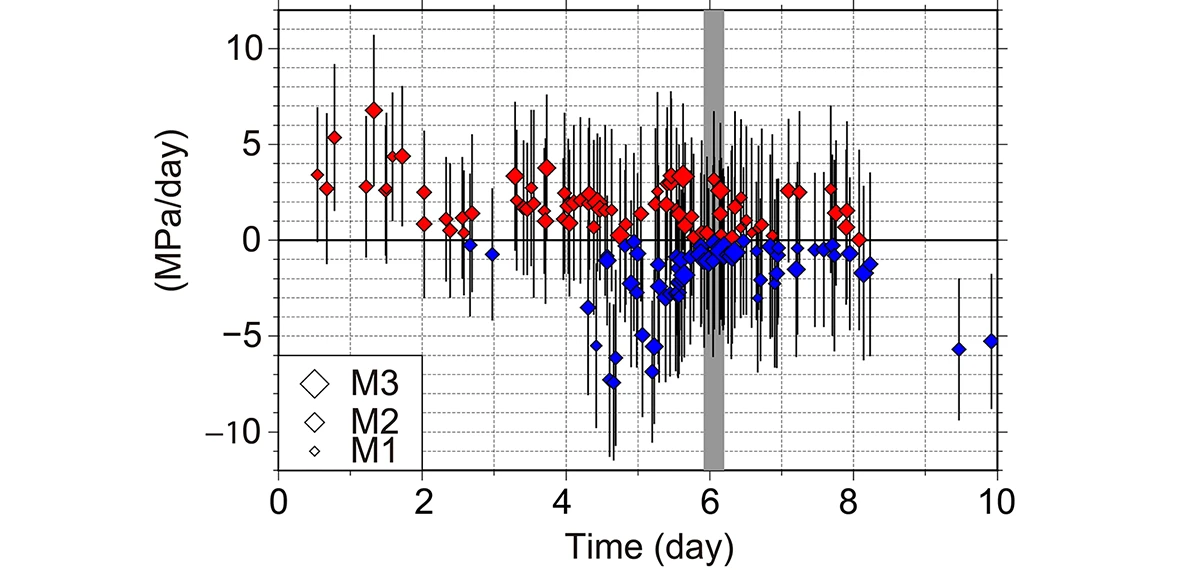
Fig. 3: The time derivative of the pore-fluid pressure field at hypocenters and at origin times.

The red and blue diamonds denote the positive and negative changes in pore-fluid pressures, respectively.

Interaction between earthquakes and volcanoes

Mt. Ontake is the second highest stratovolcano in Japan and the closest active volcano to our Nagoya University. On September 27, 2014, around 11:52 hours JST (UTC +9), Mt. Ontake produced a hydrothermal (steam-type) eruption with a volcanic explosivity index (VEI = 2) for the first time in seven years and the fourth time since recorded history. Since volcanic activity is often accompanied by seismic activity, most of which are micro seismicity, seismic observation is one of the most typical and powerful methods to examine volcanoes.

We estimated focal mechanisms of volcano-tectonic earthquakes (VT events) and demonstrated that there were significant temporal changes in types of focal mechanisms caused by volcanic activity in the two weeks before the 2014 eruption. For the precursory period, we observed many VT events of E-W tension that were not consistent with the regional stress field of strike-slip type with NW-SE compression, presumably caused by the inflation of magmatic/hydrothermal fluids, which was removed immediately after the expulsion of volcanic ejecta. The deviation of focal mechanisms from the local stress field can be an indicator of increases in volcanic activity. The proposed method may contribute to the mitigation of volcanic hazards.

Fig. 4: Seismicity around Mt. Ontake (August 2014 to March 2015).
a. Earthquake hypocenters and seismic stations in the Ontake region (The light blue squares denote seismic stations organized by Nagoya University at that time).
b. Earthquake hypocenters beneath the summit region.
c. Histogram of VT events per day in the summit region.

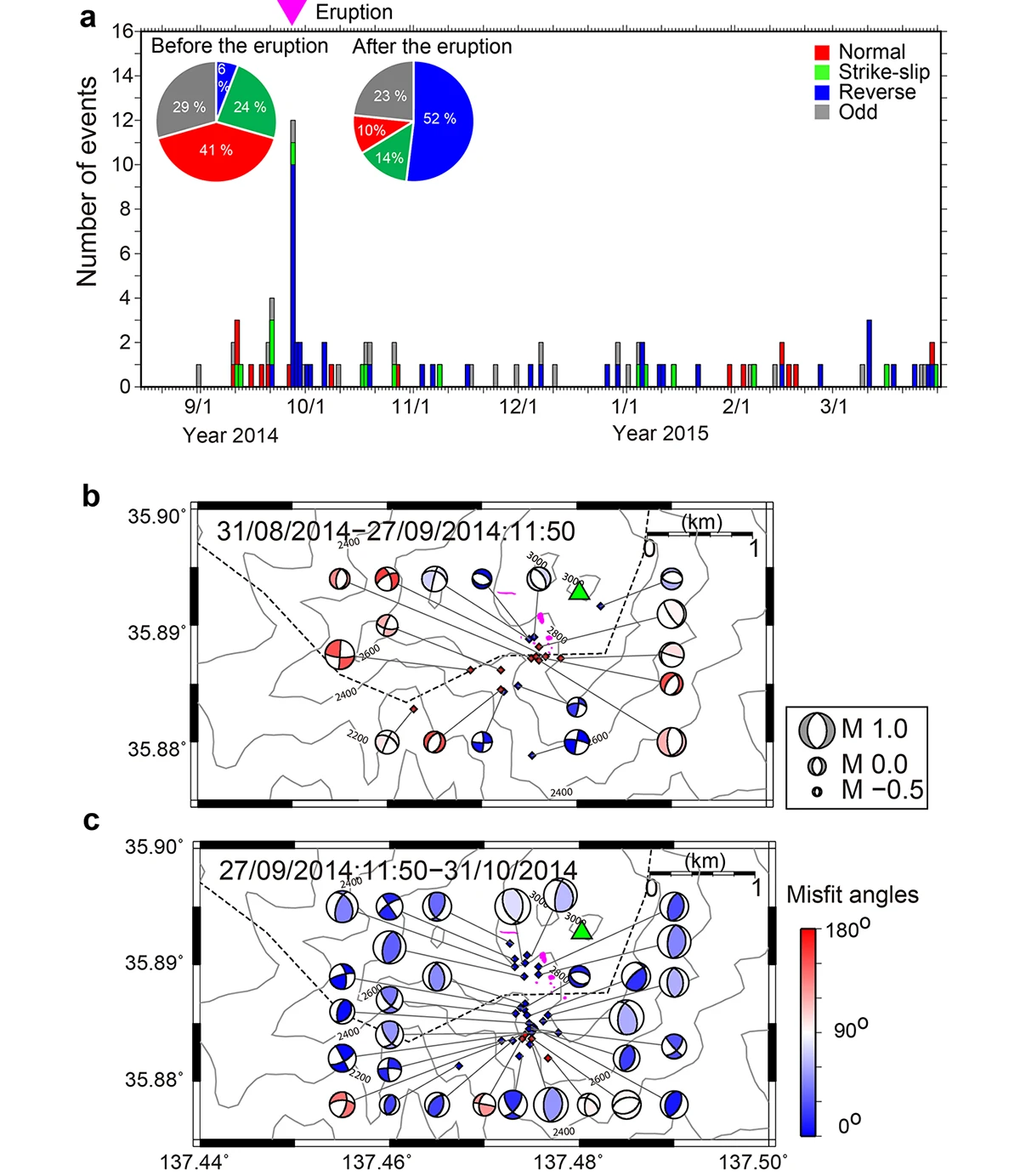
Fig. 5: Temporal changes in focal mechanism solutions.
a. Classification of focal mechanisms solutions.
b. Focal mechanism solutions for the precursory period.
c. Focal mechanism solutions for the posy-eruption period.

The color scales indicate misfit anglew between observed and theoretical slip vectors expected from the regional stress field. Pink spots mark the craters of the 2014 eruption estimated by GSI.